Chapter 21 - Areas Theorems on Parallelograms Exercise Ex. 21.1
Question 1
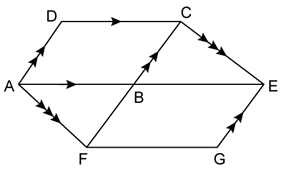
In
the given figure, if AB ∥ DC ∥
FG and AE is a straight line. Also, AD ∥ FC. Prove that:
area of ∥
gm ABCD = area of ∥ gm BFGE.
Solution 1
Joining AC and FE,
we get
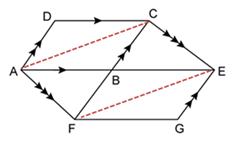
ΔAFC and ΔAFE
are on the same base AF and between the same parallels AF and CE.
⇒ A(ΔAFC)
= A(ΔAFE)
⇒ A(ΔABF)
+ A(ΔABC)
= A(ΔABF)
+ A(ΔBFE)
⇒ A(ΔABC)
= A(ΔBFE)
⇒ 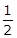 A(parallelogram ABCD) =
A(parallelogram ABCD) = 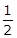 A(parallelogram BFGE) ⇒
A(parallelogram ABCD) = A(parallelogram BFGE)
A(parallelogram BFGE) ⇒
A(parallelogram ABCD) = A(parallelogram BFGE)
Question 2
In
the given figure, the perimeter of parallelogram PQRS is 42 cm. Find the
lengths of PQ and PS.
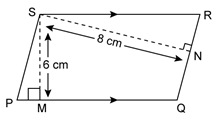
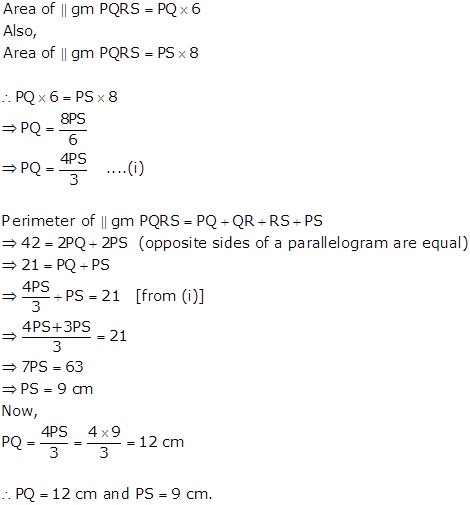
Solution 2
Question 3
In
the given figure, PT ∥ QR and QT ∥
RS. Show that: area of ΔPQR = area of ΔTQS.
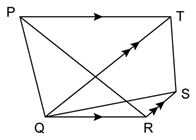
*Question
modified
Solution 3
Joining TR, we
get
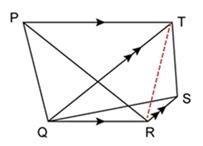
ΔPQR and ΔQTR
are on the same base QR and between the same parallel lines QR and PT.
ΔQTR and ΔTQS
are on the same base QT and between the same parallel lines QT and RS.
From (i) and (ii), we get
Question 4
In
the given figure, ΔPQR is right-angled
at P. PABQ and QRST are squares on the side PQ and hypotenuse QR. If PN ⊥
TS, show that:
(a) ΔQRB ≅
ΔPQT
(b) Area of square PABQ = area of
rectangle QTNM.
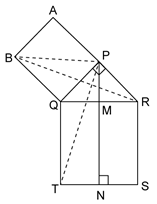
Solution 4

Question 5
The
diagonals of a parallelogram ABCD intersect at O. A line through O meets AB
in P and CD in Q. Show that
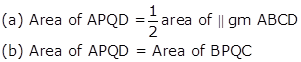
Solution 5

Question 6
In
the given figure AF = BF and DCBF is a parallelogram. If the area of ΔABC
is 30 square units, find the area of the parallelogram DCBF.
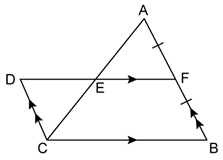
Solution 6
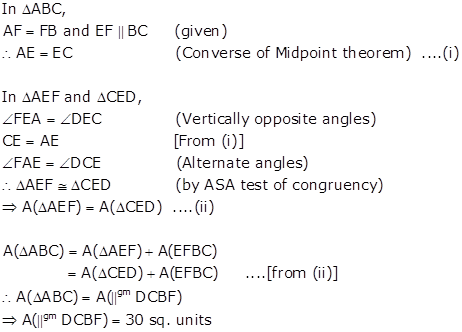
Question 7
In
the given figure, BC ∥ DE.
(a) If area of ΔADC
is 20 sq. units, find the area of ΔAEB.
(b) If the area of ΔBFD
is 8 square units, find the area of ΔCEF
Solution 7

Question 8
In the given figure, AB ∥
SQ ∥
DC and AD ∥
PR ∥
BC. If the area of quadrilateral ABCD is 24 square units, find the area of
quadrilateral PQRS.
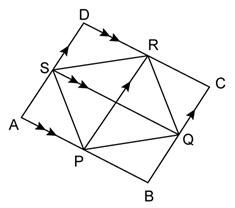
Solution 8
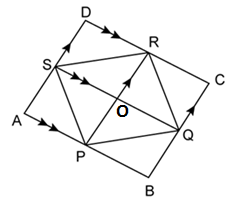
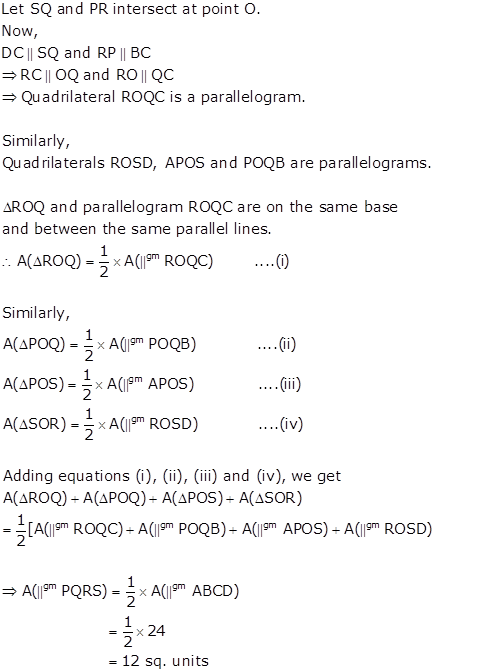
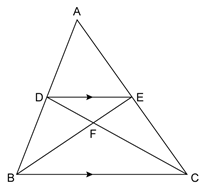
Question 9
In
the given figure, PQ ∥ SR ∥
MN, PS ∥
QM and SM ∥
PN. Prove that:
ar.
(SMNT) = ar. (PQRS).
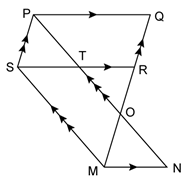
Solution 9
SM ∥
PN
⇒ SM ∥
TN
Also, SR ∥
MN
⇒ ST ∥
MN
Hence, SMNT is a
parallelogram.
SM ∥
PN
⇒ SM ∥
PO
Also, PS ∥
QM
⇒ PS ∥
OM
Hence, SMOP is a
parallelogram.
Now,
parallelograms SMNT and SMOP are on the same base SM and between the same
parallels SM and PN.
∴ A(parallelogram SMNT) =
A(parallelogram SMOP) ….(i)
Similarly, we
can show that quadrilaterals PQRS is a parallelogram.
Now,
parallelograms PQRS and SMOP are on the same base PS and between the same
parallels PS and QM.
∴ A(parallelogram PQRS) =
A(parallelogram SMOP) ….(ii)
From (i) and (ii), we have
A(parallelogram
SMNT) = A(parallelogram PQRS)
Question 10
In
the given figure, ABC is a triangle and AD is the median.
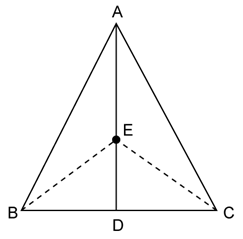
If
E is any point on the median AD. Show that:
Area
of ΔABE
= Area of ΔACE.
Solution 10
AD is the median
of ΔABC.
Therefore it will
divide ΔABC
into two triangles of equal areas.
∴ Area(ΔABD)
= Area(ΔACD) ….(i)
Similarly, ED is
the median of ΔEBC.
∴ Area(DEBD)
= Area(DECD) ….(ii)
Subtracting
equation (ii) from (i), we have
Area(ΔABD)
- Area(ΔEBD)
= Area(ΔACD)
- Area(ΔECD)
⇒ Area(ΔABE)
= Area(ΔACE)
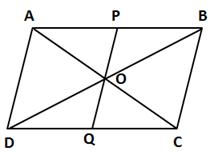
Question 11
In
the given figure, ABC is a triangle and AD is the median.

If
E is the midpoint of the median AD, prove that:
Area
of ΔABC
= 4 ×
Area of ΔABE
Solution 11
AD is the median
of ΔABC.
Therefore it
will divide ΔABC into two triangles of equal areas.
∴ Area(ΔABD)
= Area(ΔACD) ….(i)
Similarly, ED is
the median of ΔEBC.
∴ Area(ΔEBD)
= Area(ΔECD) ….(ii)
Subtracting
equation (ii) from (i), we have
Area(ΔABD)
- Area(ΔEBD)
= Area(ΔACD)
- Area(ΔECD)
⇒
Area(ΔABE)
= Area(ΔACE) ….(iii)
Since E is the mid-point
of median AD,
AE = ED
Now,
ΔABE
and ΔBED
have equal bases and a common vertex B.
∴
Area(ΔABE)
= Area(ΔBED) ….(iv)
From (i),
(ii), (iii) and (iv), we get
Area(ΔABE)
= A(ΔBED)
= Area(ΔACE)
= Area(ΔEDC) ….(v)
Now,
Area(ΔABC)
= Area(ΔABE)
+ A(ΔBED)
+ Area(ΔACE)
+ Area(ΔEDC)
= 4 ×
Area(ΔABE) [From (v)]
Question 12
In
a parallelogram PQRS, M and N are the midpoints of the sides PQ and PS
respectively. If area of ΔPMN is 20 square
units, find the area of the parallelogram PQRS.
Solution 12
Construction: Join SM and SQ.

In a parallelogram PQRS, SQ is
the diagonal.
So, it bisects the parallelogram.
∴
Area(DPSQ)

SM is the median of ΔPSQ.
∴
Area(ΔPSM)
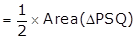
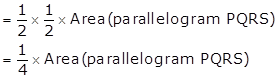
Again, MN is the median of ΔPSM.
∴
Area(ΔPMN)
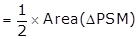
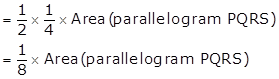
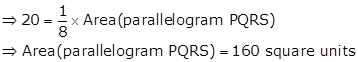
Question 13
In
a parallelogram PQRS, T is any point on the diagonal PR. If the area of ΔPTQ
is 18 square units find the area of ΔPTS.
Solution 13
Construction: Join
QR. Let the diagonals PR and QS intersect each other at point O.
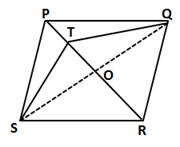
Since diagonals
of a parallelogram bisect each other, therefore O is the mid-point of both PR
and QS.
Now, median of a
triangle divides it into two triangles of equal area.
In ΔPSQ,
OP is the median.
∴ Area(ΔPOS)
= Area(ΔPOQ) ….(i)
Similarly, OT is
the median of ΔTSQ.
∴Area(ΔTOS)
= Area(ΔTOQ) ….(ii)
Subtracting
equation (ii) from (i), we have
Area(ΔPOS)
- Area(ΔTOS)
= Area(ΔPOQ)
- Area(ΔTOQ)
⇒
Area(ΔPTQ)
= Area(ΔPTS)
⇒
Area(ΔPTS)
= 18 square units
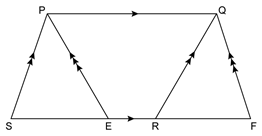
Question 14
In
the given figure area of ∥ gm PQRS is 30 cm2. Find the height of ∥
gm PQFE if PQ = 6 cm.
Solution 14
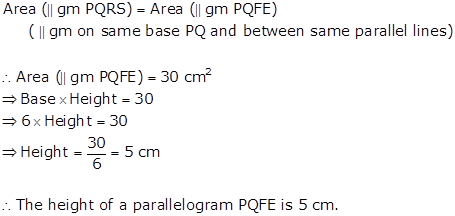
Question 15
In
the given figure, PQRS is a ∥ gm. A straight
line through P cuts SR at point T and QR produced at N. Prove that area of
triangle QTR is equal to the area of triangle STN.

Solution 15
ΔPQT
and parallelogram PQRS are on the same base PQ and between the same parallel
lines PQ and SR.
ΔPSN
and parallelogram PQRS are on the same base PS and between the same parallel
lines PS and QN.
Adding equations (i) and (ii), we get
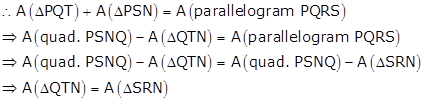
Subtracting A(ΔRTN)
from both the sides, we get
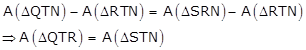
Question 16
In
the given figure, ST ∥ PR. Prove that:
area of quadrilateral PQRS = area of ΔPQT.
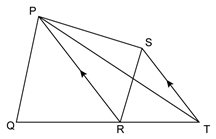
Solution 16
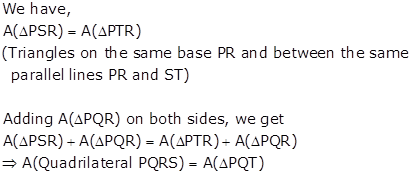
Question 17
Solution 17
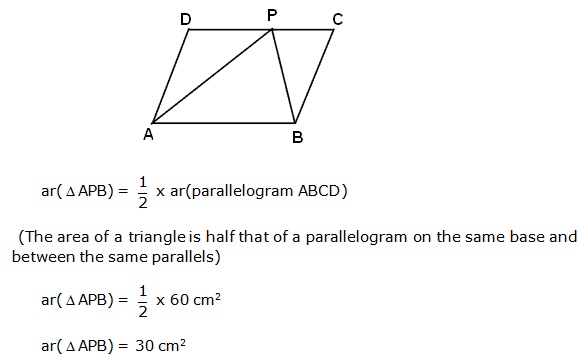
Question 18
Solution 18
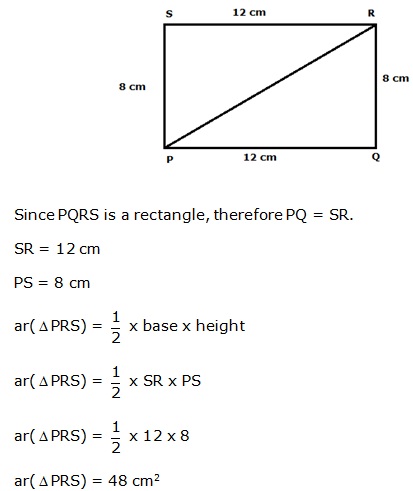
Question 19
Solution 19


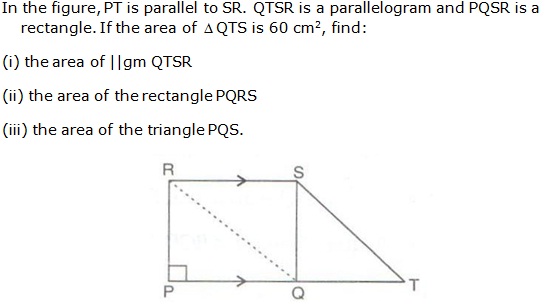
Question 20

Solution 20
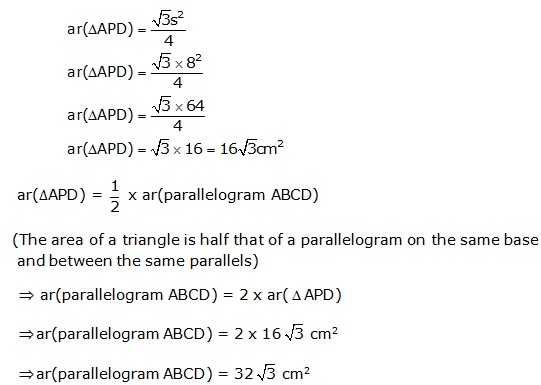
Question 21
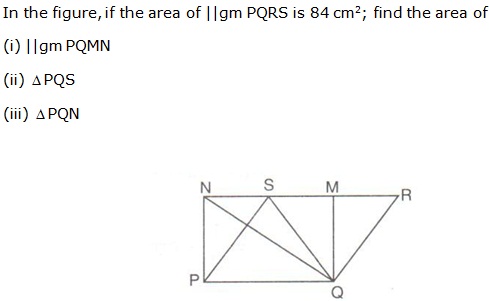
Solution 21

Question 22
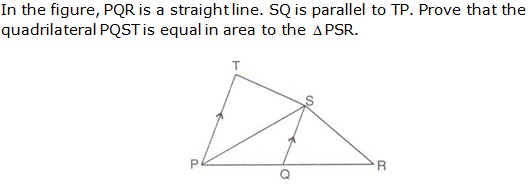
Solution 22

Question 23
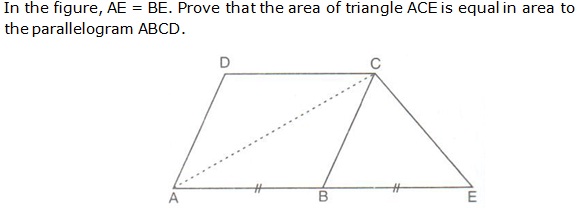
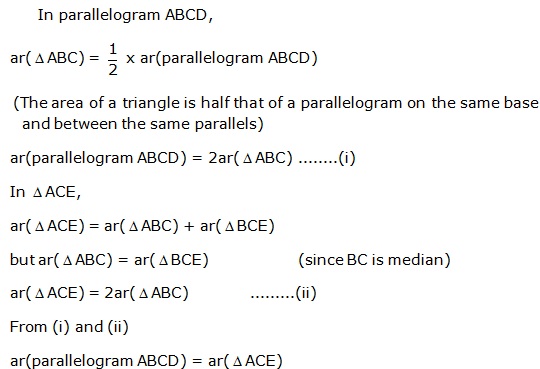
Solution 23
Question 24
Solution 24

Question 25
Solution 25
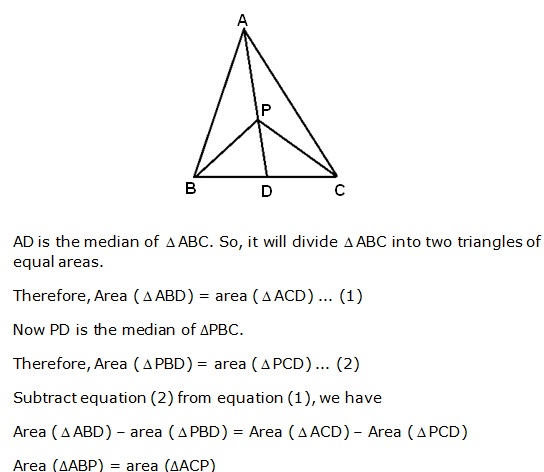
Question 26
Solution 26
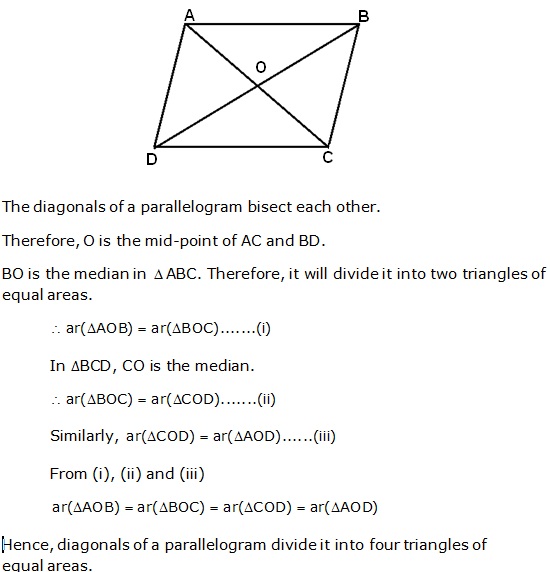
Question 27
Solution 27
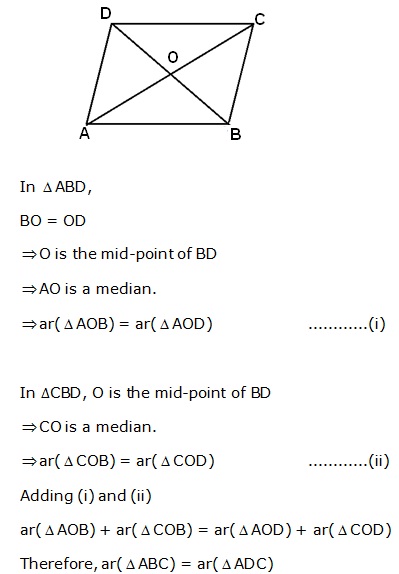
Question 28
Solution 28

Question 29

Solution 29
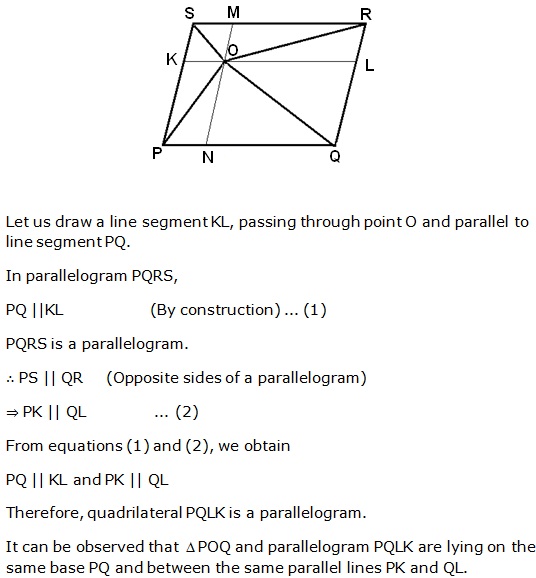
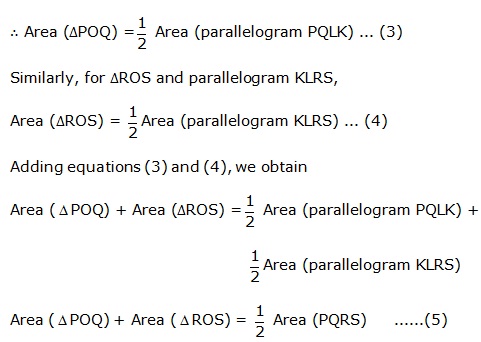
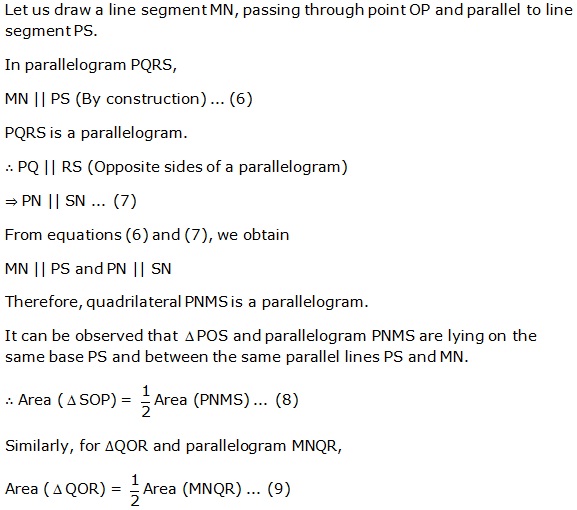

Question 30
Solution 30
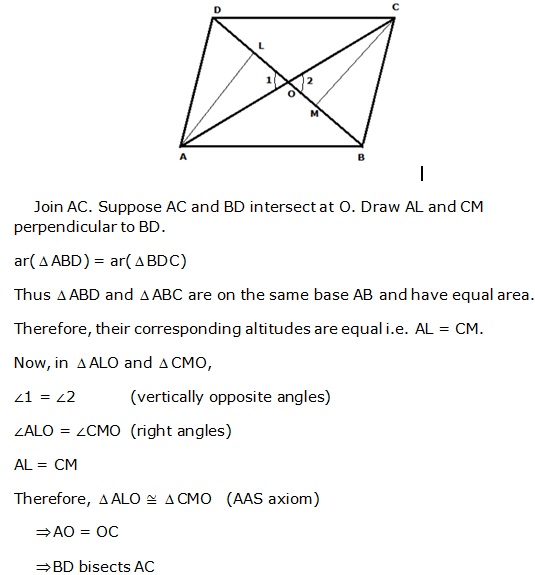
Question 31

Solution 31
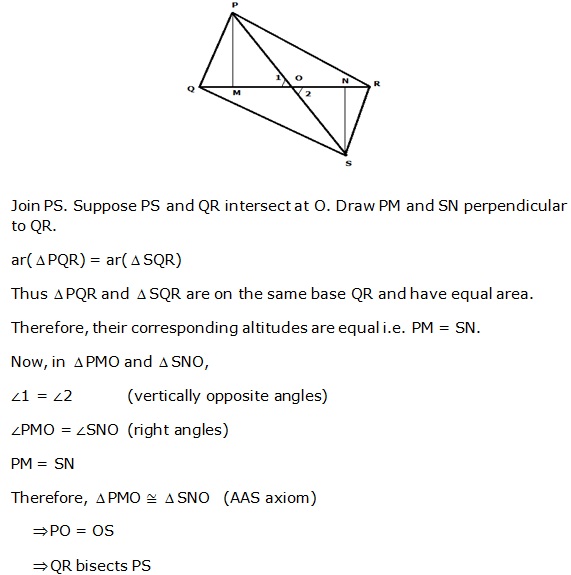
Question 32
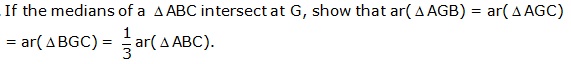
Solution 32
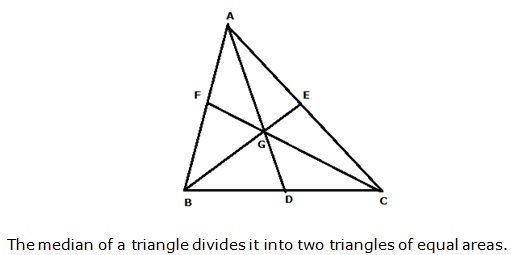
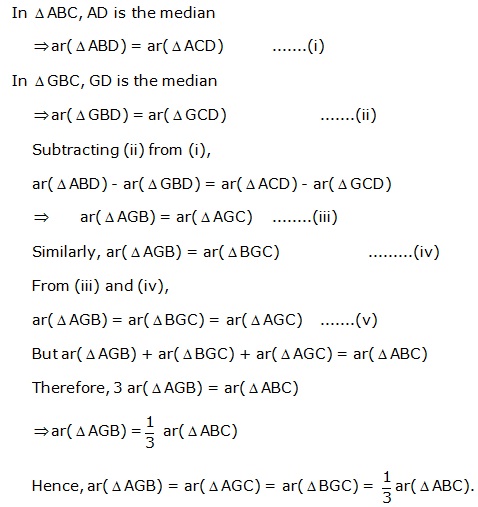
Question 33
Solution 33
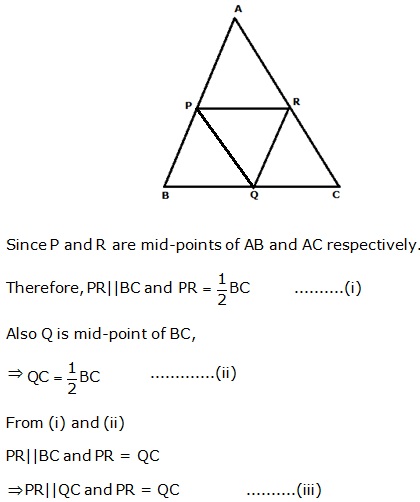
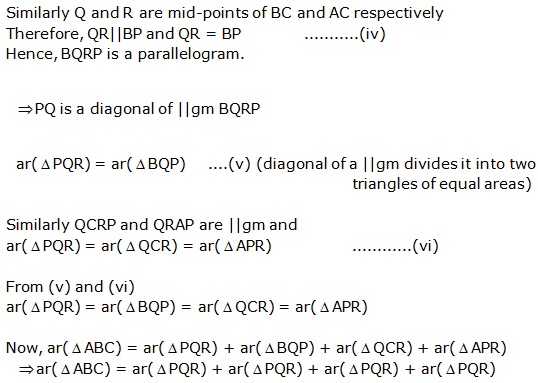

Question 34

Solution 34


Question 35
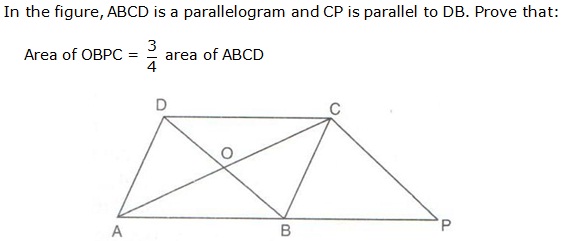
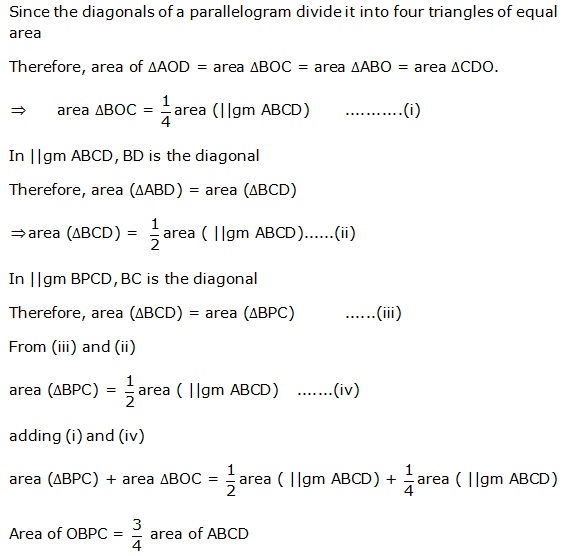
Solution 35
Question 36
Solution 36

Question 37
Solution 37



0 comments:
Post a Comment