FRANK Solutions for Class 9 Maths Chapter 22 - Statistics
Chapter 22 - Statistics Exercise Ex. 22.1
Question 1
Solution 1

Question 2

Solution 2

Question 3

Solution 3
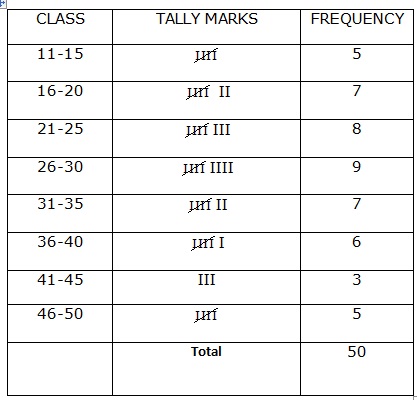

Question 4

Solution 4

Question 5
Solution 5
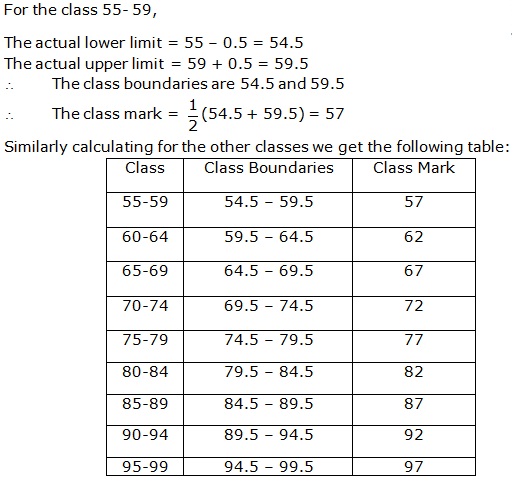
Question 6
Find
the actual (or true) lower and upper class limits and class-marks (or mid
values) of the following classes: 2.1 - 4.0, 4.1 - 6.0 and 6.1 - 8.0.
Solution 6

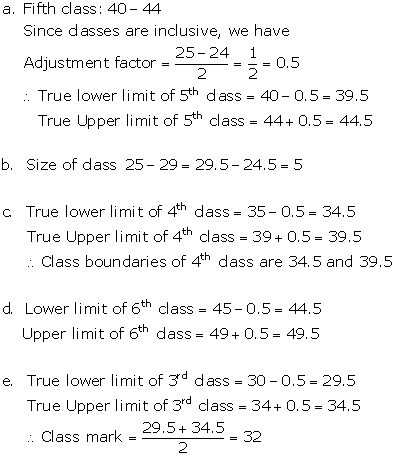
Question 7
Observe
the given frequency table to answer the following:
Class Interval
|
20-24
|
25-29
|
30-34
|
35-39
|
40-44
|
45-49
|
Frequency
|
6
|
12
|
10
|
15
|
9
|
2
|
a. The true class limits of the
fifth class.
b. The size of the second class.
c. The class boundaries of the
fourth class.
d. The upper and lower limits of
the sixth class.
e. The class mark of the third class.
Solution 7
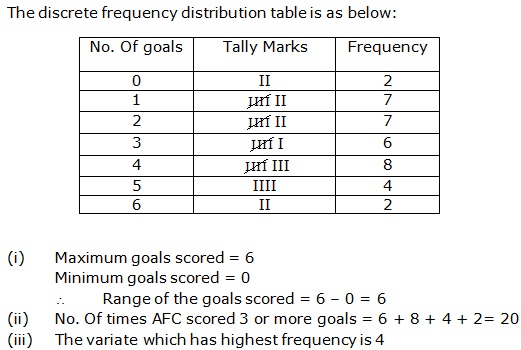
Question 8

Solution 8
Question 9

Solution 9

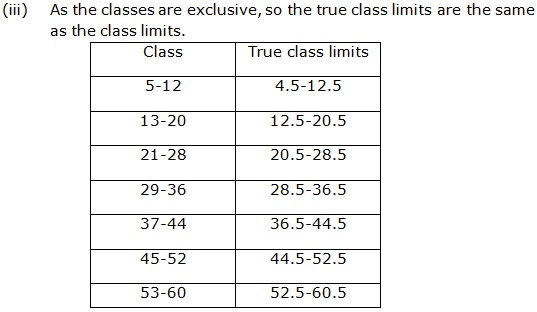
Question 10
Solution 10
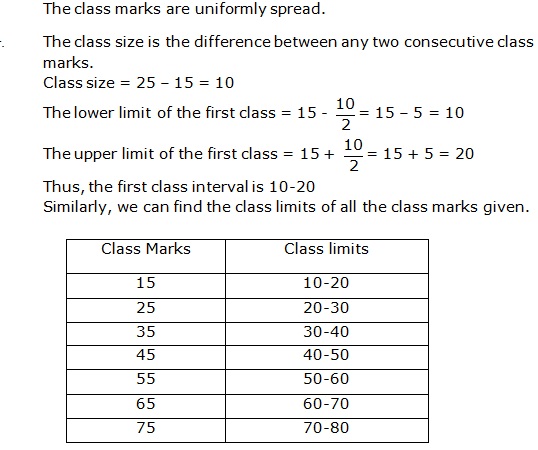
Question 11
Solution 11

Question 12

Solution 12

Chapter 22 - Statistics Exercise Ex. 22.2
Question 1
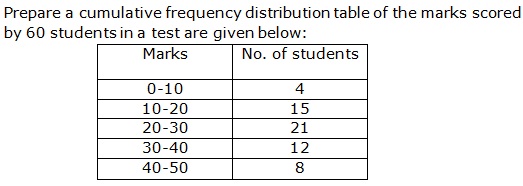
Solution 1
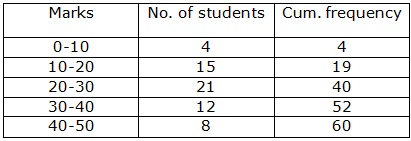
Question 2
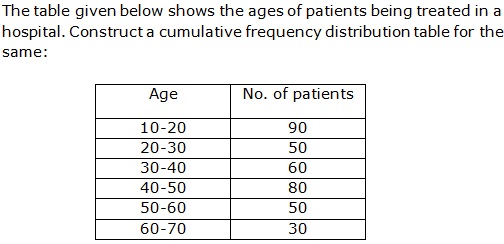
Solution 2

Question 3

Solution 3
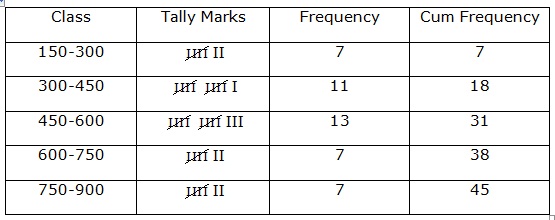
Question 4
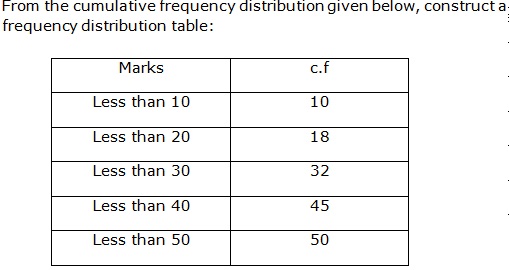
Solution 4
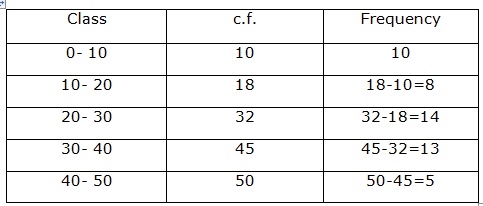
Question 5
Construct
a frequency distribution table from the given cumulative frequency
distribution showing the weights of 750 students in a school:
Weight (in kg)
|
c.f.
|
More Than 25
|
750
|
More Than 30
|
640
|
More Than 35
|
615
|
More Than 40
|
485
|
More Than 45
|
370
|
More Than 50
|
220
|
More Than 55
|
124
|
More Than 60
|
49
|
More Than 65
|
24
|
More Than 70
|
0
|
a. Find the number of students
whose weight lie in the interval 40-45
b. Find the interval which has the
most number of students.
Solution 5
Frequency distribution table is
as follows:
Weight
(in kg)
|
c.f.
|
25
- 30
|
110 (750 - 640)
|
30
- 35
|
25 (640 - 615)
|
35
- 40
|
130 (615 - 485)
|
40
- 45
|
115 (485 - 370)
|
45
- 50
|
150 (370 - 220)
|
50
- 55
|
96 (220 - 124)
|
55
- 60
|
75 (124 - 49)
|
60
- 65
|
25 (49 - 25)
|
65
- 70
|
24 (24 - 0)
|
70
- 75
|
0
|
a. The number of students whose
weight lie in the interval 40 - 45 is 115.
b. The interval 45 - 50 has the
most number of students.
Chapter 22 - Statistics Exercise Ex. 22.3
Question 1

Solution 1
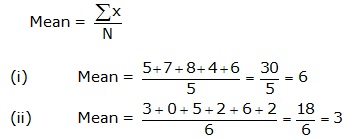
Question 2
Solution 2

Question 3
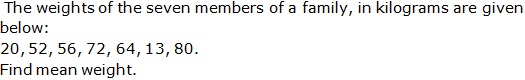
Solution 3
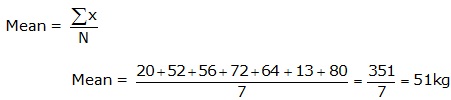
Question 4
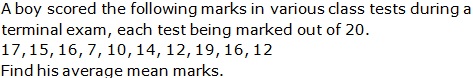
Solution 4
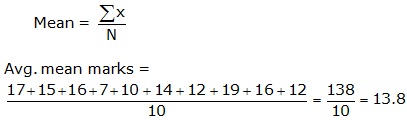
Question 5
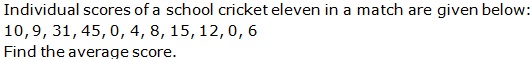
Solution 5
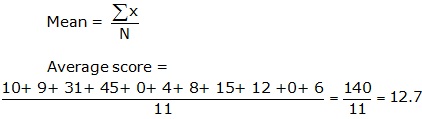
Question 6
The
daily maximum relative humidity (in per cent) in Mumbai from May 1 to May 7,
1992 is given below:
64, 70, 65, 80, 75, 78
Find the mean.
Solution 6

Question 7

Solution 7
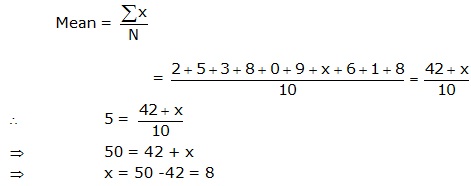
Question 8
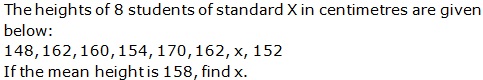
Solution 8
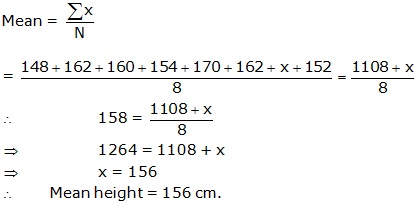
Question 9
Solution 9
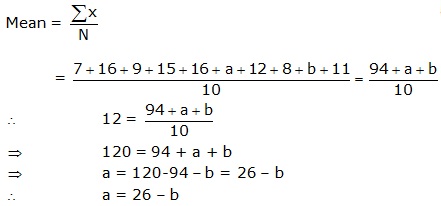
Question 10

Solution 10

Question 11

Solution 11

Question 12
The
mean of 16 natural numbers is 48. Find the resulting mean, if each of the
number is
a. increased by 5
b. decreased by 8
c. multiplied by 4
d. divided by 0.25
e. increased by 50%
f. decreased by 10%
Solution 12

Question 13
The
mean of 4 observations is 20. If one observation is excluded, the mean of the
remaining observations becomes 15. Find the excluded observation.
Solution 13

Question 14
The
mean monthly income of 8 men is Rs. 8079.75. A man whose monthly income
is Rs. 8280 has
also been taken into consideration. Calculate the mean monthly income of all
the men.
Solution 14
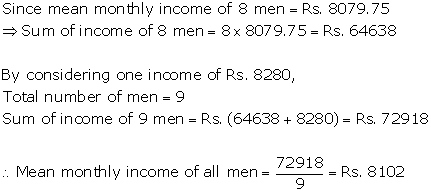
Question 15
The
mean of 200 observations is 20. It is found that the value of 180 is wrongly
copied as 280. Find the actual mean.
Solution 15

Question 16

Solution 16
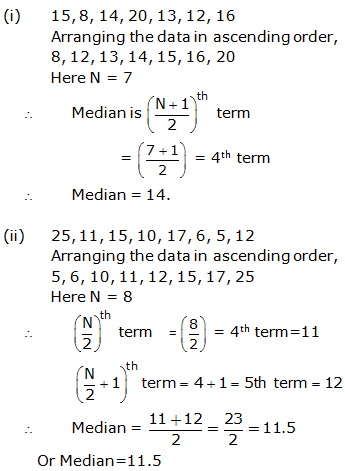
Question 17
Solution 17
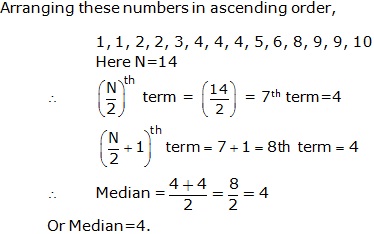
Question 18
Solution 18

Question 19
The following data has been arranged in ascending order.
0, 1, 2, 3, x + 1, x + 5, 20, 21, 26, 29.
Find the value of x, if the median is 5.
Solution 19

Question 20

Solution 20


0 comments:
Post a Comment