Chapter 28 - Coordinate Geometry Exercise Ex. 28.1
Question 1
Find the value of 'a' and 'b' if
a. (a + 2,5 + b) = (1, 6)
b. (2a + b, a - 2b) = (7, 6)
Solution 1
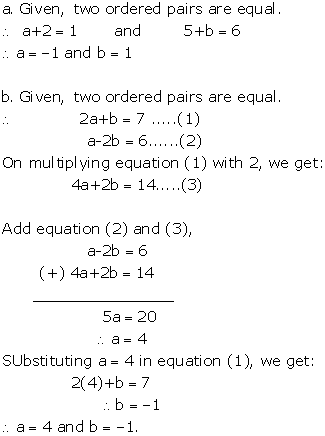
Question 2
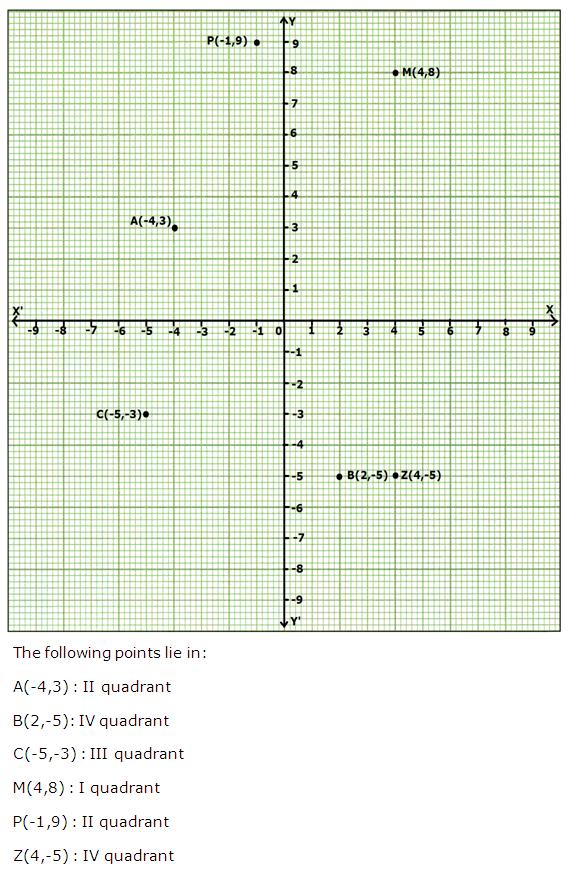
Solution 2
Question 3
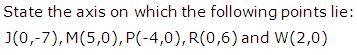
Solution 3
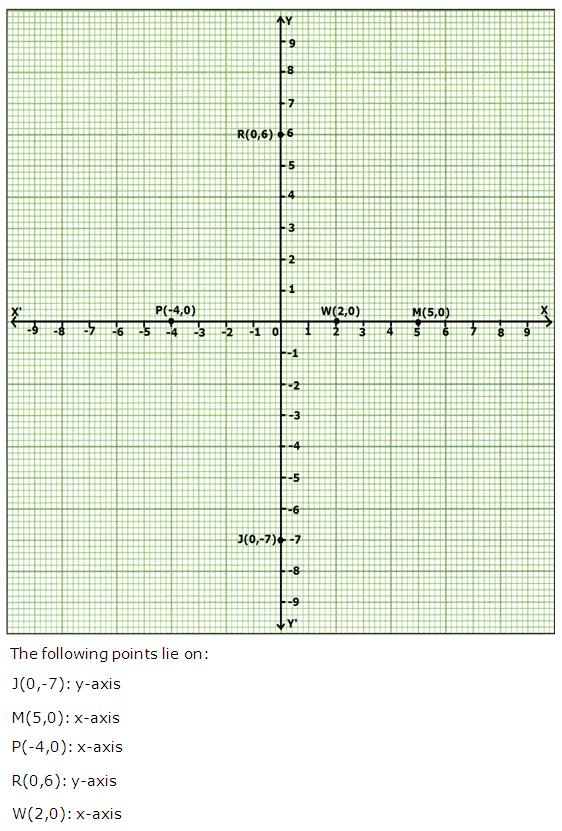
Question 4

Solution 4

Question 5
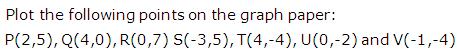
Solution 5
Question 6
Plot the points O (0, 0), P (6, 0) and R
(0, 5) on a graph. Find the coordinates of the point Q such that OPQR is a
rectangle.
Solution 6
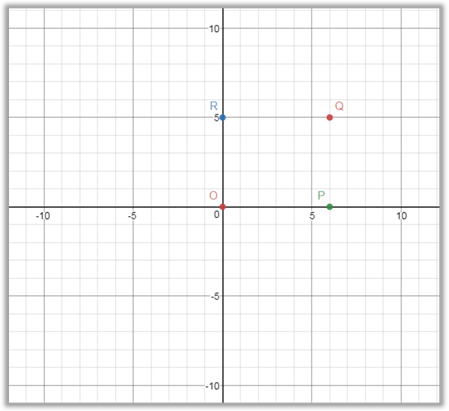
Q=(6,5)
Question 7
Plot the points A (3, 4) and C (-3, -2)
on a graph. Find the coordinates of the point B and D such ABCD is a square.
Also find the area of the square.
Solution 7
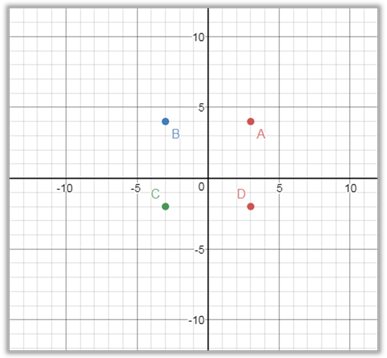
B=(-3,4)
D=(3,-2)

Question 8
Plot the points (-2, 3), (3, 3), (5, -2)
and (-5, -2) on a graph and join them in order. Name the figure you get.
Solution 8
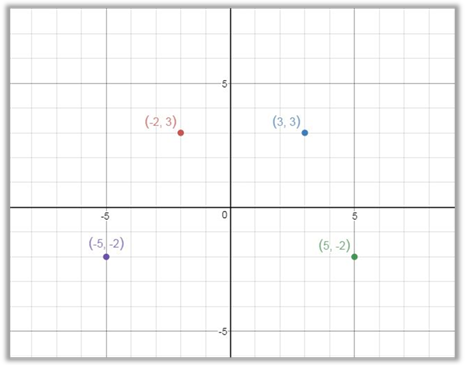
The quadrilateral obtained is a
trapezium.
Question 9
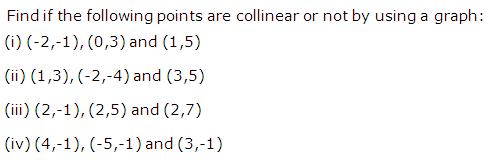
Solution 9
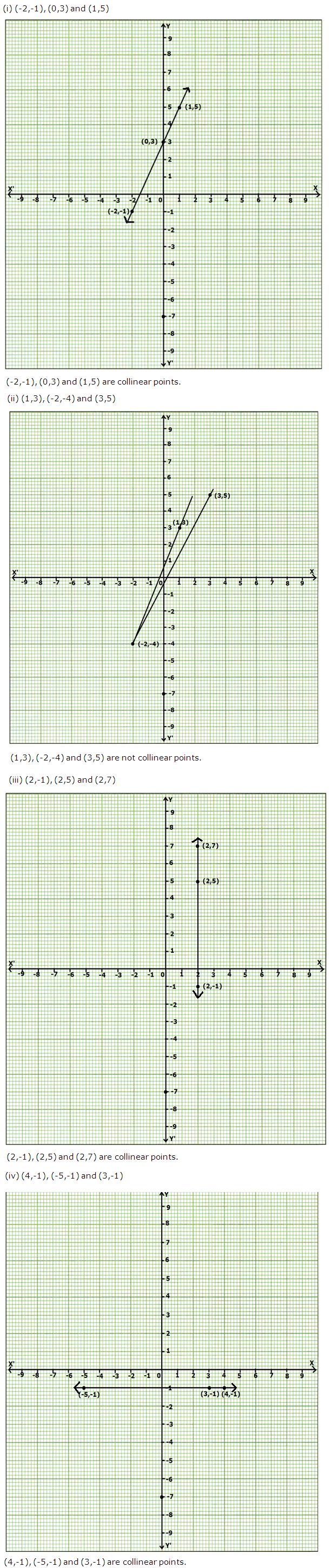
Question 10
In each of the following, find the
coordinates of the point whose abscissa is the solution of the first equation
and ordinate is the solution of the second equation.
Solution 10
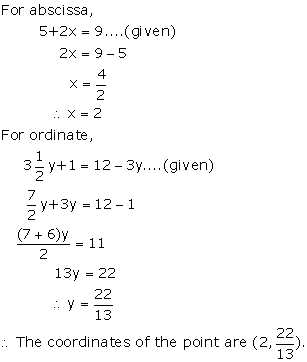
Question 11
In each of the following, find the
coordinates of the point whose abscissa is the solution of the first equation
and ordinate is the solution of the second equation.
Solution 11
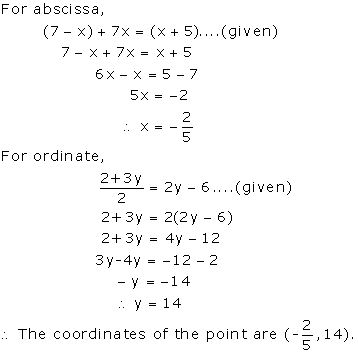
Question 12
A rectangle PQRS is drawn on the
coordinate axes such that P is the origin, PQ = 6 units and PS = 5 units.
Find the coordinates of the vertices P, Q R and S. Also, find the area of the
rectangle.
Solution 12
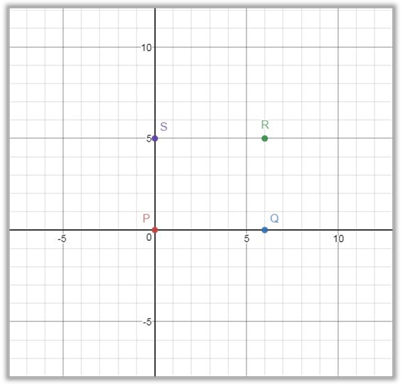
P=(0,0)
Q=(6,0)
R=(6,5)
S=(0,5)
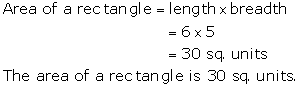
Chapter 28 - Coordinate Geometry Exercise Ex. 28.2
Question 1
Express the equation 3x + 5y + 15 = 0 in
the form such that:
a. x is subject to the formula
b. y is dependent variable and x is
independent variable.
Solution 1
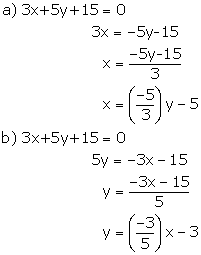
Question 2
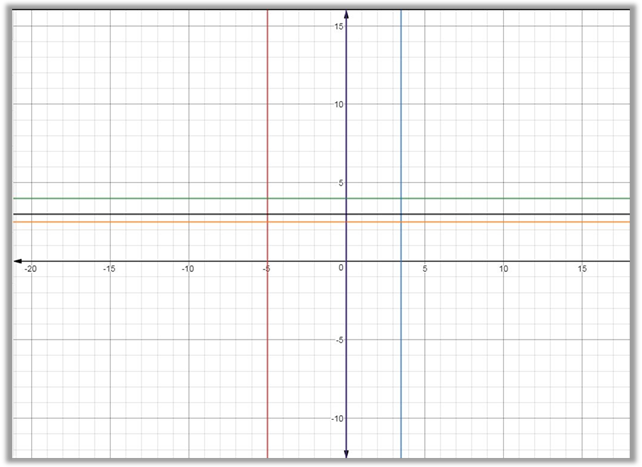
Draw a graph of each of the following
equations:
a. x + 5 = 0
b. y - 4 = 0
c. 2x = 7
d. 2y - 5 = 0
e. x = 0
f. y = 3
Solution 2

Question 3
Draw a graph of each of the following
equations:
a. x + 6y = 15
b. 3x - 2y = 6
c. 3y + 2x = 11
d. 5x + 2y = 16
e. x + y - 3 = 0
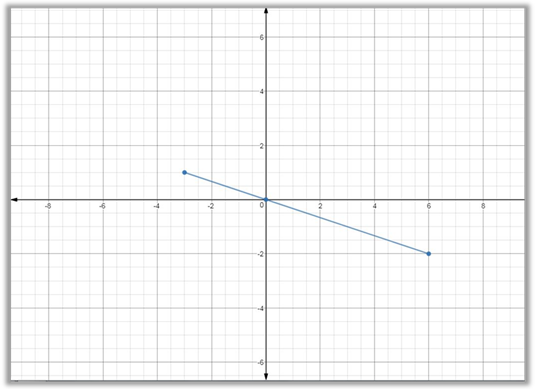
f. x = 3y
g. 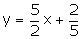
h. 
i. 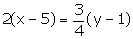
j. 
Solution 3
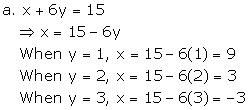
x
|
9
|
3
|
-3
|
y
|
1
|
2
|
3
|
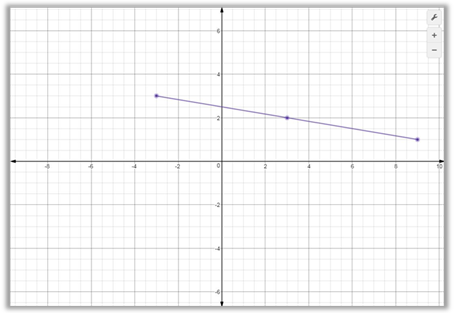

x
|
2
|
4
|
-2
|
y
|
0
|
3
|
-6
|

x
|
1
|
-2
|
-5
|
y
|
3
|
5
|
7
|

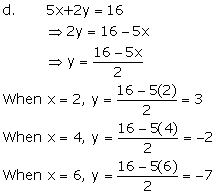
x
|
2
|
4
|
6
|
y
|
3
|
-2
|
-7
|
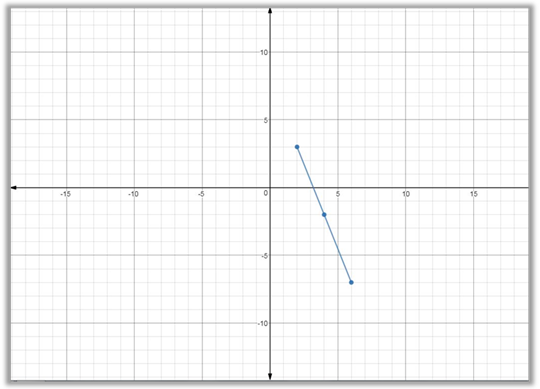
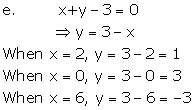
x
|
2
|
0
|
6
|
y
|
1
|
3
|
-3
|
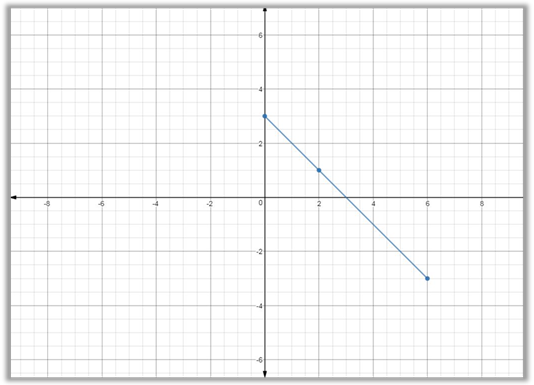
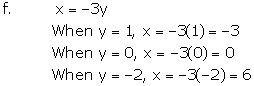
x
|
-3
|
0
|
6
|
y
|
1
|
0
|
-2
|
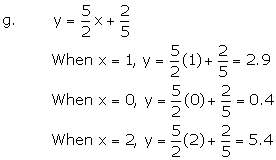
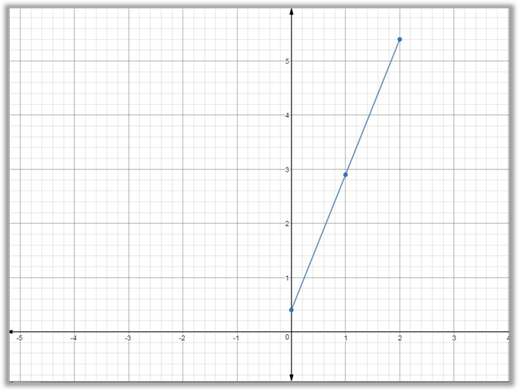
x
|
1
|
0
|
2
|
y
|
2.9
|
0.4
|
5.4
|
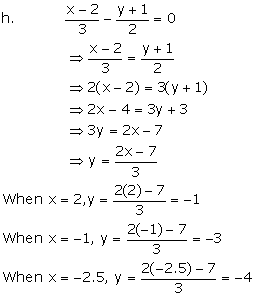
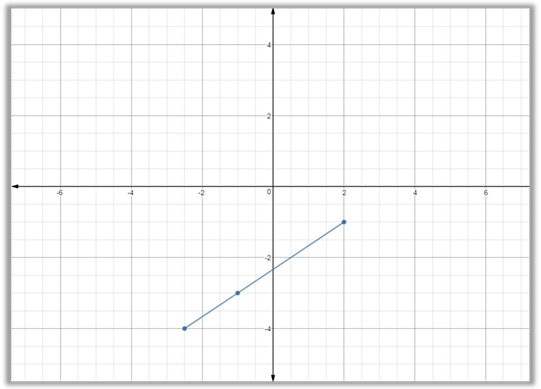
x
|
2
|
-1
|
-2.5
|
y
|
-1
|
-3
|
-4
|
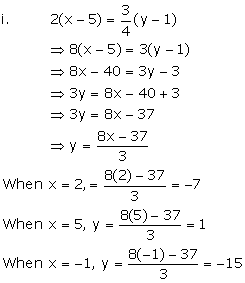
x
|
2
|
5
|
-1
|
y
|
-7
|
1
|
-15
|
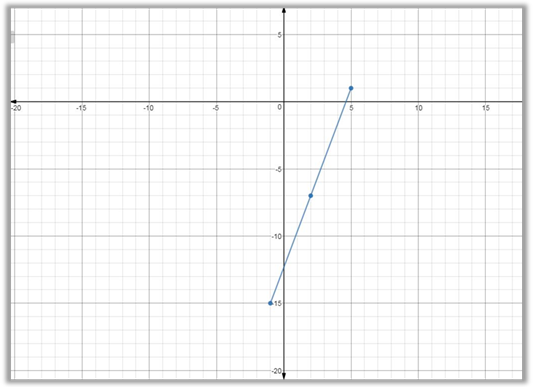
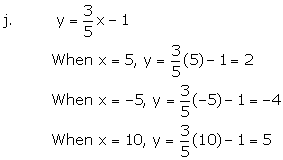
x
|
5
|
-5
|
10
|
y
|
2
|
-4
|
5
|
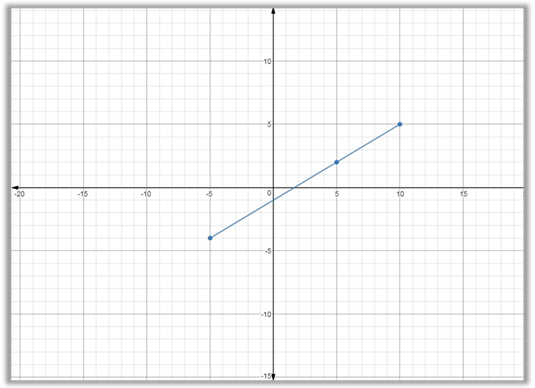
Question 4
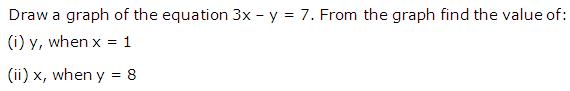
Solution 4
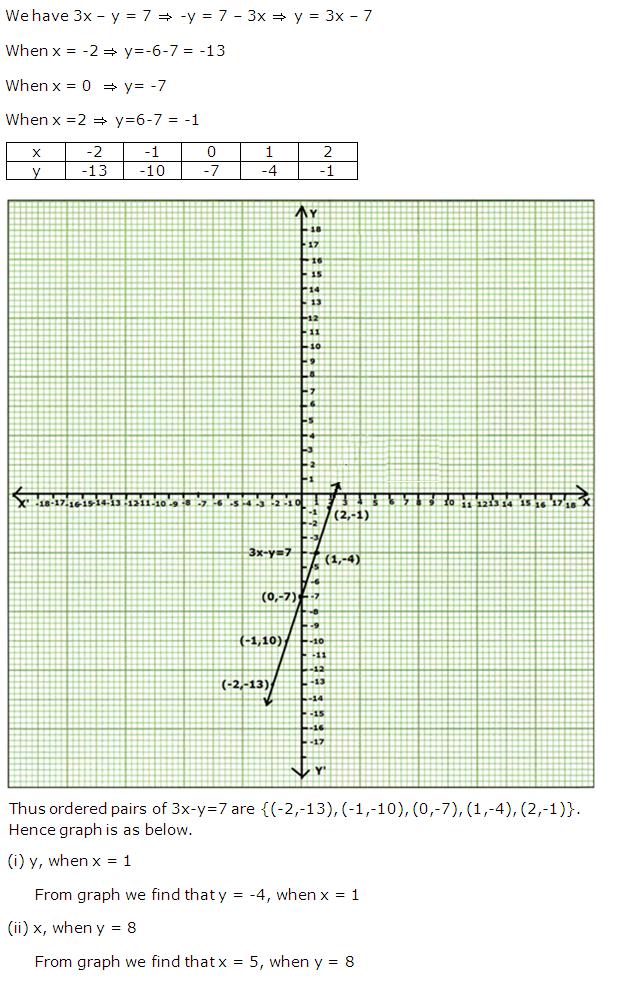
Question 5
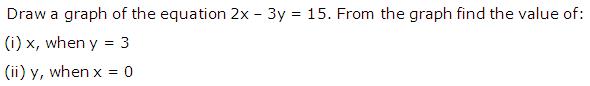
Solution 5

Question 6
Draw a graph for each of the following
equations and find the coordinates of the points where the line drawn meets
the x-axis and y-axis:
a. 2x + 3y = 12
b. 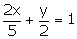
Solution 6
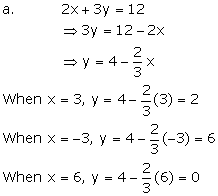
x
|
3
|
-3
|
6
|
y
|
2
|
6
|
0
|

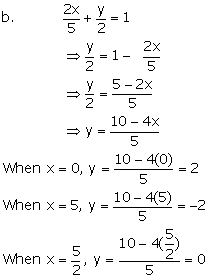
x
|
0
|
5
|
5/2
|
y
|
2
|
-2
|
0
|
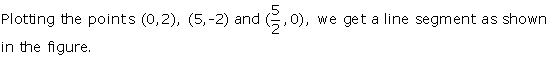
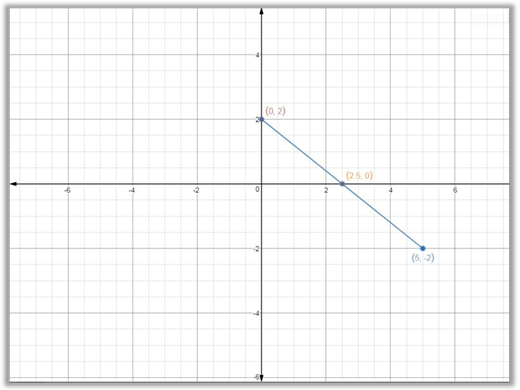
Question 7

Solution 7

Question 8
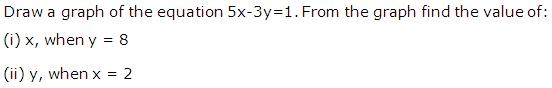
Solution 8
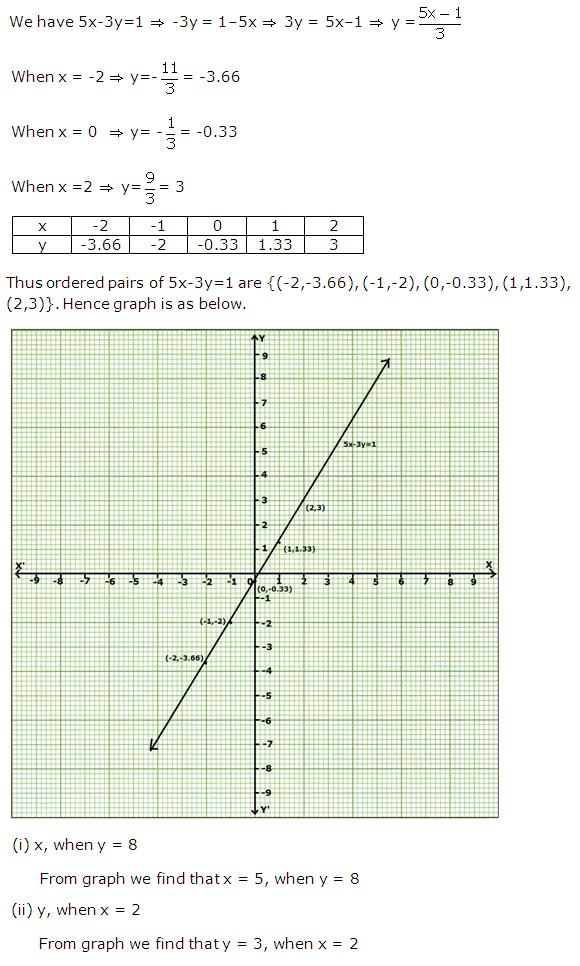
Question 9
Draw the graph of the lines y = x + 2, y
= 2x - 1 and y = 2 from x = -3 to 4, on the same graph paper. Check whether
the lines drawn are parallel to each other.
Solution 9

x
|
0
|
5
|
-3
|
y
|
2
|
7
|
-1
|
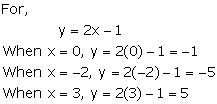
x
|
0
|
-2
|
3
|
y
|
-1
|
-5
|
5
|
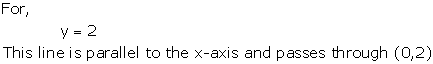
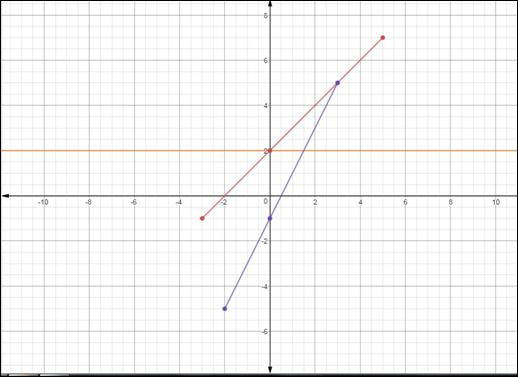
The lines are not parallel to each other.
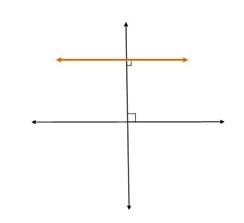
Question 10
Find the inclination and slope of a line
which is
a. equidistant from the x-axis.
b. equidistant from the y-axis.
c. intersecting x-axis at right angle.
d. perpendicular to y-axis.
Solution 10
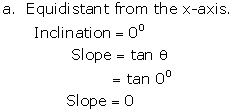

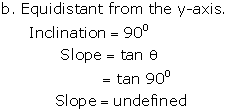
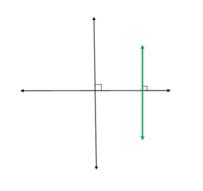
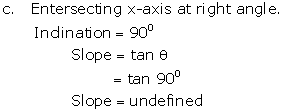
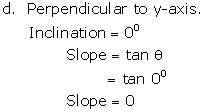
Question 11
Find the slope of the line whose
inclination is given as
a. 0°
b. 30°
c. 45°
d. 60°
Solution 11
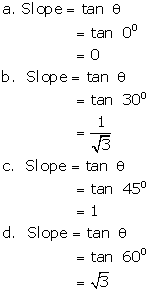
Question 12
Find the inclination of the line whose slope
is
a. 1
b. 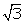
Solution 12
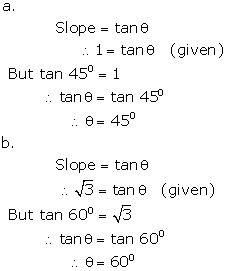
Question 13
Find the slope and y-intercept for each
of the following equations:
a. 3x - 8y + 24 = 0
b. 6x = 7y - 12
Solution 13
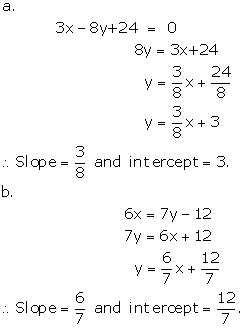
Question 14
Find the equation of the line, whose
a. slope is 3 and y-intercept is 5.
b. slope is 0 and y-intercept is -1.
c. slope is 1 and y-intercept is 0.
Solution 14

Question 15
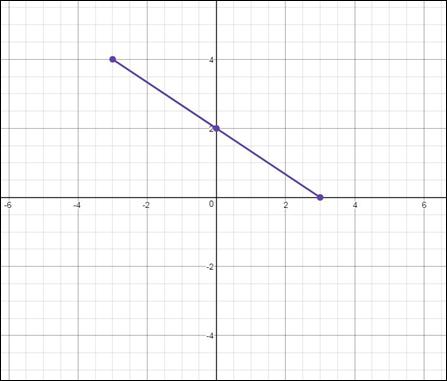
Draw the graph of a line 2x + 3y = 6.
From the graph, find the slope and y-intercept of the line.
Solution 15
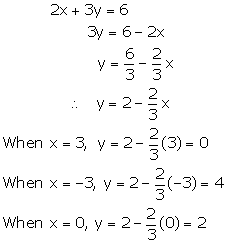
x
|
3
|
-3
|
0
|
y
|
0
|
4
|
2
|

Question 16
Draw the graph of the lines represented
by the equations x + y = 4 and 2x - y = 2 on the same graph. Find the
coordinates of the point where they intersect
Solution 16
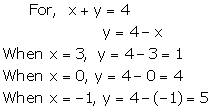
x
|
3
|
0
|
-1
|
y
|
1
|
4
|
5
|

x
|
3
|
0
|
-1
|
y
|
4
|
-2
|
-4
|
|
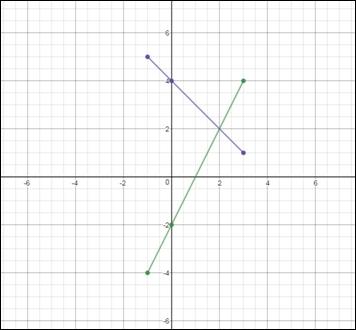
Question 17
Draw the graph of the lines represented by the equations 3x - 2y = 4 and x + y = 3 on the same graph. Find the coordinates of the point where they intersect. State, whether the lines are perpendicular to each other.
Solution 17

Question 18

Solution 18
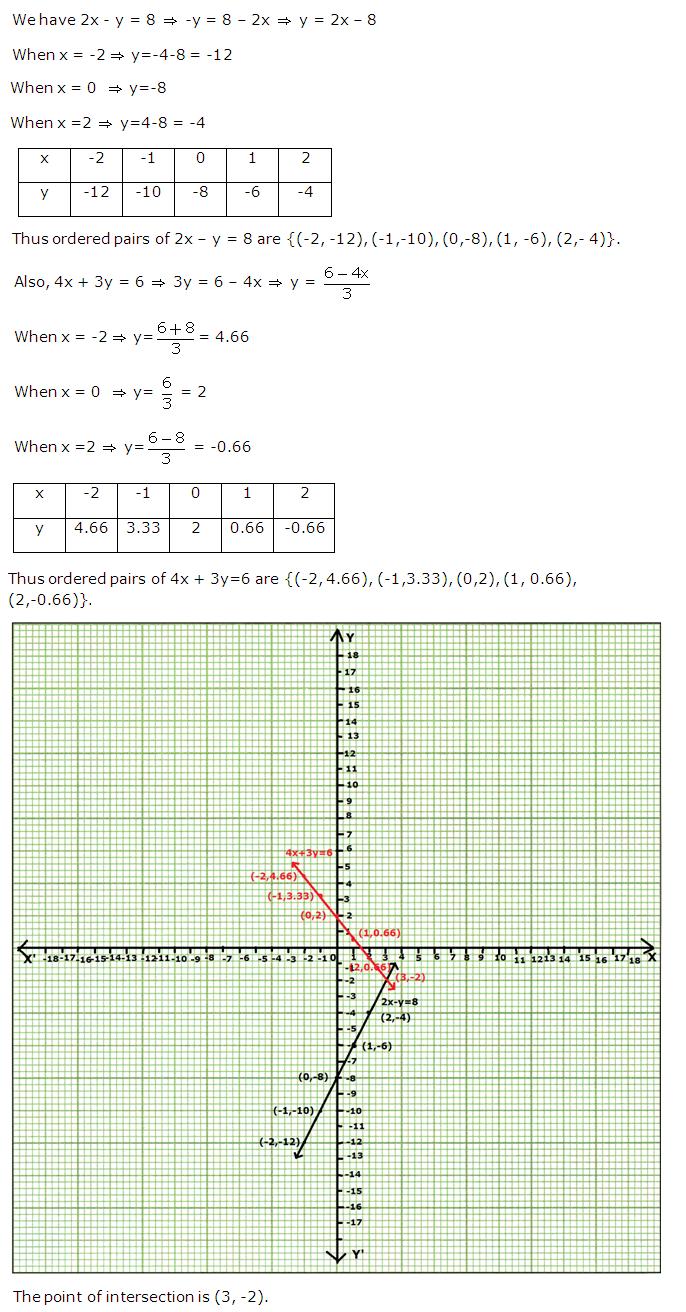
Question 19
Draw the graph of the lines represented
by the equations 5y = 3x + 1 and y = 2x + 3 on the same graph. Find the
coordinates of the point where they intersect.
Solution 19
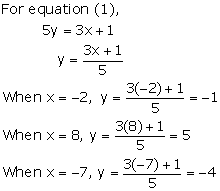
x
|
-2
|
8
|
-7
|
y
|
-1
|
5
|
-4
|
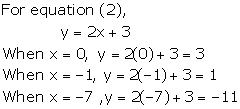
x
|
0
|
-1
|
-7
|
y
|
3
|
1
|
-11
|
|


0 comments:
Post a Comment