FRANK Solutions for Class 10 Maths Chapter 8 - Reflection

Chapter 8 - Reflection Exercise Ex. 8.1

Question 1

1. State the co-ordinates of the images of the following points under reflection in the x-axis:
(i) (3,-9)
(ii) (-7, 5)
(iii) (0, 6)
(iv) (-4,-8)

Solution 1

(i) (3,-9)
The co-ordinate of the given point under reflection in the x-axis is: (3,9).
(ii) (-7, 5)
The co-ordinate of the given point under reflection in the x-axis is: (-7,-5).
(iii) (0, 6)
The co-ordinate of the given point under reflection in the x-axis is: (0,-6).
(iv) (-4,-8)
The co-ordinate of the given point under reflection in the x-axis is: (-4, 8).

Question 2

A point P is mapped onto P' under the reflection in the x-axis. P' is mapped onto P" under the reflection in the origin. If the co-ordinates of
P" are (5,-2), write down the co-ordinates of P. State the single transformation that takes place.

Solution 2

P"= (5,-2), therefore, co-ordinates of P' = (-5, 2) and hence the coordinates of P = (-5,-2)
Single transformation = reflection in the y-axis

Question 3

Write down the co-ordinates of the image of the point (-2, 4) under reflection in the origin and under reflection in the y-axis. What is the distance between the points of reflection?

Solution 3

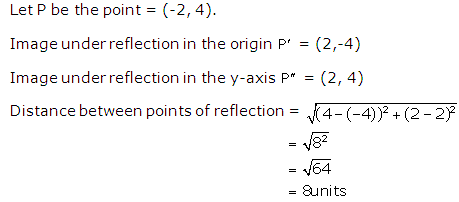
Question 4

Solution 4

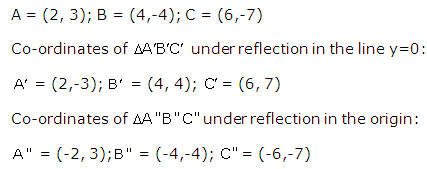
Question 5

A point P (-8, 1) is reflected in the x-axis to the point P'. The point P' is  then reflected in the origin to point P". Write down the co-ordinates of P". State the single transformation that maps P into P".

Solution 5

P = (-8, 1), therefore co-ordinates of P' under reflection in the x-axis = (-8, -1).
Hence, the co-ordinates of P" under reflection in the origin = (8, 1).
The single transformation = reflection in the y-axis.

Question 6

Solution 6

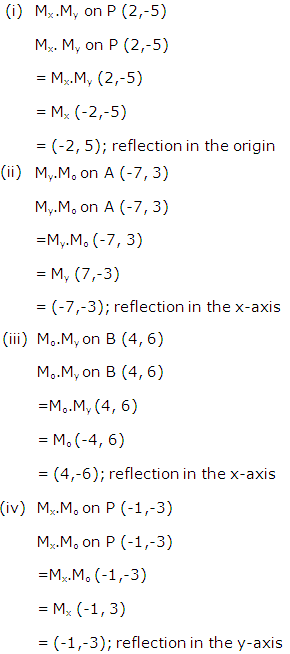
Question 7

Find the co-ordinates of the image of A (-5, 4) after reflection in the line
(i) y = 0
(ii) y = 4

Solution 7

(i) y = 0
Co-ordinates of image = (-5, 2x0-4)= (-5,-4)
(ii) y = 4
Co-ordinates of image = (-5, 2x4-4)= (-5,4)

Question 8

Find the co-ordinates of the image of S(4,-1) after reflection in the line
(i) x = 0
(ii) y = 5

Solution 8

(i) x = 0
Co-ordinates of image =(2x0-4,-1)= (-4,-1)
(ii) y = 5
Co-ordinates of image = (4, 2x5-(-1)) = (4, 11)

Question 9

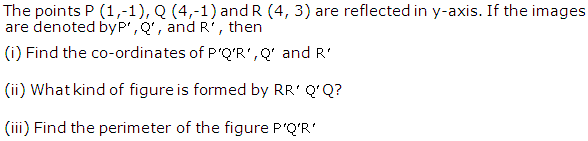
Solution 9

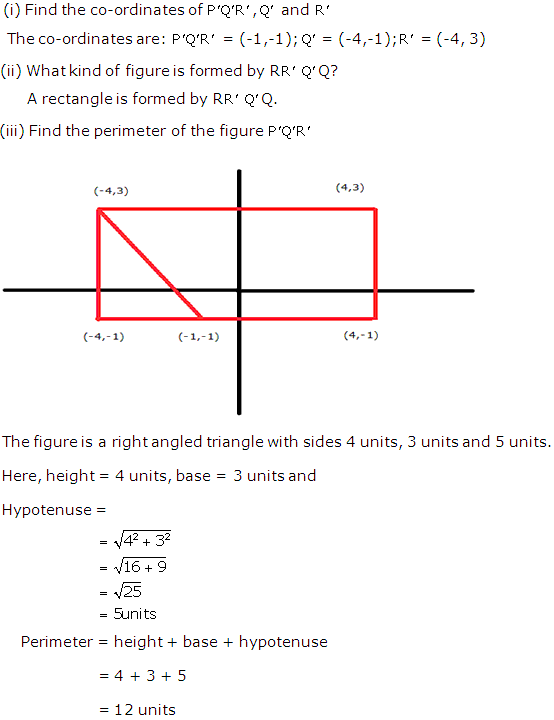
Question 10

Solution 10

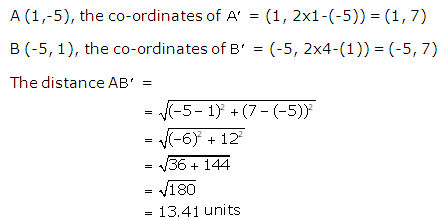
Question 11

Solution 11

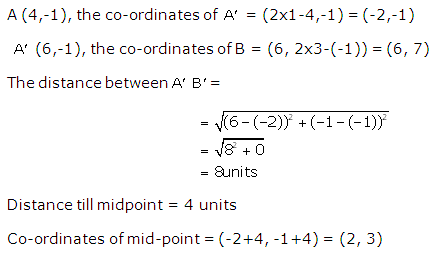
Question 12

State the co-ordinates of the images of the following points under reflection in the y-axis:
(i) (2, 8)
(ii) (-1,-3)
(iii) (5,-6)
(iv) (-4, 7)

Solution 12

(i) (2, 8)
The co-ordinate of the given point under reflection in the y-axis is: (-2,8).
(ii) (-1,-3)
The co-ordinate of the given point under reflection in the y-axis is: (1,-3).
(iii) (5,-6)
The co-ordinate of the given point under reflection in the y-axis is: (-5,-6).
(iv) (-4, 7)
The co-ordinate of the given point under reflection in the y-axis is: (4, 7).

Question 13

State the co-ordinates of the images of the following points under reflection in the origin:
(i) (-1,-4)
(ii) (2, 7)
(iii) (0, 2)
(iv) (9,-9)

Solution 13

(i) (-1,-4)
The co-ordinate of the given point under reflection in the origin is: (1, 4)
(ii) (2, 7)
The co-ordinate of the given point under reflection in the origin is:  (-2,-7)
(iii) (0, 2)
The co-ordinate of the given point under reflection in the origin is: (0,-2)
(iv) (9,-9)
The co-ordinate of the given point under reflection in the origin is:  (-9, 9)

Question 14

P' is the image of P under reflection in the x-axis. If the co-ordinates of P' are (2, 10), write the co-ordinates of P.

Solution 14

P' = (2, 10). Therefore, the co-ordinates of P under reflection in the x-axis = (2,-10)

Question 15

S' is the image of S under reflection in the origin. If the co-ordinates of S are (2,-5), write the co-ordinates of S'.

Solution 15

S= (2,-5). Therefore, the co-ordinates of S' under reflection in the origin = (-2, 5)

Question 16

A point P is reflected in the x-axis to P'. P' is then reflected in the origin to P". If the co-ordinates of P' are (-3, 4). Find the co-ordinates of P and P". Write the single transformation that map P onto P".

Solution 16

P' = (-3, 4).
Therefore, the co-ordinates of P under reflection in the x-axis = (-3,-4)
and the co-ordinates of P" under reflection in the origin = (3,-4).
The single transformation = reflection in the y-axis.

Question 17

A point P is reflected in the x-axis. Co-ordinates of its image are (8,-6). Find the co-ordinates of P. Find the co-ordinates of the image of P under reflection in the y-axis.

Solution 17

P' = (8,-6).
Therefore, the co-ordinates of P under reflection in the x-axis = (8, 6)
and the co-ordinates of P" under reflection in the y-axis = (-8, 6).

Question 18

A point R (3,-2) is reflected in the origin as R'. Point Q (-7, 1) is reflected in the x-axis as Q'. Write down the co-ordinates of R' and Q'. Calculate the distance R' Q'.

Solution 18

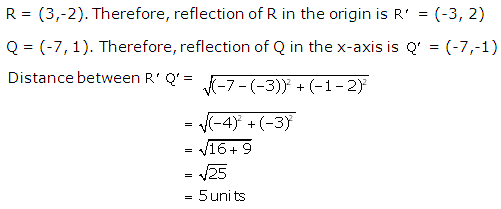
Question 19

The points B and C have the co-ordinates (3, 2) and (0, 3). Find B', the image of B under the reflection in the x-axis and C', the image of C under the reflection in the line BB'.

Solution 19

B = (3, 2), Therefore, reflection of B in the x-axis is B'= (3,-2)
C = (0, 3), Therefore, reflection of C in the line B  is C' = (6, 3).